|
|
Optimal parameters of a sinusoidal
representation of signals
A. Kocsor, L. Toth, I.
Balint
In the spectral analysis of digital signals, one of the
most useful parametric models is the representation by a sum of phase-shifted
sinusoids in form of 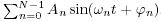 , where , where  and and
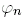 are the component’s amplitude, frequency and
phase, respectively. This model generally fits well speech and most musical
signals due to the shape of the representation functions. If using all
of the above parameters, a quite di±cult optimization problem arises.
The applied methods are generally based on eigenvalue decomposition [3].
However this procedure is computationally expensive and works only if
the sinusoids and the residual signal are statistically uncorrelated.
To speed up the representation process also rather ad hoc methods occur
[4]. The presented algorithm applies the newly established Homogeneous
Sinus Representation Function (HSRF) to find the best representing subspace
of fixed dimension N by a BFGS optimization. The optimum parameters are the component’s amplitude, frequency and
phase, respectively. This model generally fits well speech and most musical
signals due to the shape of the representation functions. If using all
of the above parameters, a quite di±cult optimization problem arises.
The applied methods are generally based on eigenvalue decomposition [3].
However this procedure is computationally expensive and works only if
the sinusoids and the residual signal are statistically uncorrelated.
To speed up the representation process also rather ad hoc methods occur
[4]. The presented algorithm applies the newly established Homogeneous
Sinus Representation Function (HSRF) to find the best representing subspace
of fixed dimension N by a BFGS optimization. The optimum parameters
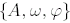 ensure the mean square error of approximation to be below
a preset threshold. ensure the mean square error of approximation to be below
a preset threshold.
|
|





